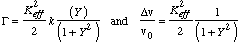(1)A.Tilke, C. Rocke, and A.Wixforth
Sektion Physik der LMU, Geschwister Scholl-Platz 1, D-80539 München
Germany
(Received )
A quantitative analysis of the frequency dependent acousto-electric interaction between a high mobility quasi-two dimensional electron system in a semiconductor heterostructure and a surface sound wave propagating on a piezoelectric crystal is presented. We show that for this hybrid system a residual air gap between both partners strongly affects the acousto-electric interaction. Frequency dependent measurement of the interaction enables us to determine the influence of the air gap on the strength of interaction and relevant parameters for the theoretical description. Our analysis might be of importance for the design of future acousto-electric experiments but also devices where strong interaction between the sound wave and the low dimensional electron system is required.
The interaction between surface acoustic waves and low-dimensional electron systems as present in semiconductor heterojunctions has attracted considerable interest over the past years [1..4]. This interaction has proven to be a powerful tool for the investigation of the dynamical conductivity [[sigma]]([[omega]],k) of the low-dimensional electron system especially in high magnetic fields where quantum effects become important. Surface acoustic waves (SAW) of wavelengths in the (sub-) micron regime are propagated through the semiconductor containing the low-dimensional electron system where the mutual interaction leads to giant quantum oscillations in the propagation parameters of the wave that directly reflect the dynamical conductivity of the electron system. Particular examples are investigations of both the integer [1] and the fractional quantum Hall effect [2] under the presence of a surface acoustic wave. SAW transmission experiments as well as acousto-electric effects [5] being related to a phonon drag have been the subject of recent studies. One particular example for the power of this remarkable technique is the discovery of a new type of quasi-particles [3] , the so-called composite Fermions that nowadays are believed to be responsible for the fractional quantum Hall effect. Recently, also experiments using quasi-one dimensional electron systems [6] and quantum point contacts [7] have been reported and revealed new and exciting aspects of the acousto-electric effects in low-dimensional electron systems.
For the experiments as described above, the usual arrangement is such that the sound wave and the electron system under investigation are present on the same (piezoelectric) semiconductor substrate like, e.g., GaAs. The interaction between the SAW and a quasi-two dimensional electron system (Q2DES) as confined in a semiconductor heterojunction could be shown to be of relaxation type. The SAW attenuation and the renormalization of the sound velocity caused by the interaction turned out to be given by 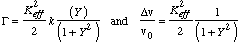[1]:
(1)
where Y:=[[sigma]]/[[sigma]]m and [[sigma]]m=vo([[epsilon]]o+[[epsilon]]s) ~
3.5.10-7[[Omega]]-1 for GaAs denotes a
critical conductivity where maximum attenuation
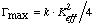occurs. k = 2[[pi]]/[[lambda]] is the wave vector of the SAW , vo the
sound velocity for a free surface, and [[epsilon]]S and [[epsilon]]0 are the
dielectric permittivities of the substrate and free space, respectively. The
material parameter
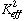=6.4.10-4
for (100)-cut GaAs and a [110] propagating SAW determines the strength
of the interaction. From (1) it is evident that the interaction is strongest
for very small sheet conductivities [[sigma]] ~ [[sigma]]m , indicating the
importance for experiments in the quantum Hall regime. However, usual
semiconductor substrates are only weak piezoelectrics such that the achievable
interaction is only a weak effect, and a piezoelectric substrate with
considerably higher
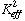would
be desired for strong interaction between the sound wave and the electron
system.
As pointed out earlier, this acousto-electric interaction is also a valuable
tool for the non-destructive and contactless characterization of a
semiconductor sample using a proximity coupling scheme as described in refs.
[8, 9]. One such system is for instance (YZ-cut) LiNbO3, which provides an
electro-mechanical coupling coefficient
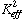=0.048
and thus bringing the strength of the interaction into even technologically
interesting regions. The major problem, however, using the proximity coupling
technique is the existence of a residual airgap between the piezoelectric and
the semiconductor heterojunction under investigation.
Here, we would like to present our recent investigations regarding the influence of such a residual airgap on the strength of the interaction and the relevant parameters that are important for its description. For this purpose, we used a state-of-the-art multi-frequency SAW delay line on (YZ-cut) LiNbO3 substrates on which a pair of split-4 interdigital transducers [10,11] provided a whole set of operating frequencies between f=100MHz and f=2.35 GHz on the same sample. Both GaAs/AlxGa1-xAs (sample B) as well as AlSb/InAs/AlSb (samples A and C) semiconductor structures containing high mobility Q2DES are pressed face down on the piezoelectric to study the interaction in this sandwich system. As the technique described works on most any semiconductor structures, a detailed description of the systems examined is omitted here for the sake of clarity. Experimentally, the sandwich is located in the center of a superconducting solenoid providing magnetic fields up to B=15 Tesla at low temperatures T > 2 K. Short RF pulses ([[tau]] ~ 1usec) at the desired operating frequency are supplied to one of the transducers and the transmitted SAW signal as a function of the applied magnetic field is analyzed in terms of intensity and sound velocity as described in detail in ref. [1] using standard boxcar techniques.
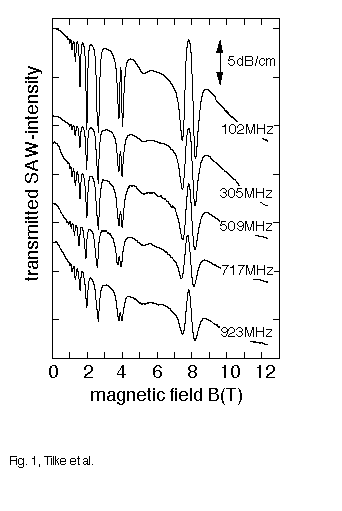
In Fig. 1, we depict a set of experimentally obtained transmission spectra for
different SAW frequencies as a function of the magnetic field. Clearly, the
above mentioned giant quantum oscillations reflecting the Shubnikov - deHaas
oscillations of the magneto conductivity [[sigma]]xx(B) are observed. A
characteristic fingerprint of the interaction between SAW and Q2DES is the
splitting of the quantum oscillations at high magnetic fields [1]. Such a
splitting arises whenever the magneto conductivity [[sigma]]xx(B) falls below
the value of [[sigma]]m, i.e. in the regime of the quantized Hall effect. The
major observation in Fig. 1 is that this splitting of the high-field
oscillations decreases with increasing frequency. At the same time the maximum
attenuation which occurs when [[sigma]]xx(B)=[[sigma]]m decreases with
increasing frequency. The characteristic lineshape can only be well described
using eq. (1) if one takes into account a sandwich-specific coupling constant
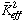and
a modified critical conductivity
.
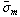Both quantities should obviously depend on the frequency or more specifically
on the product k.d where d is the width of the airgap. This
dependence is caused by the fact that the time varying electric fields
accompanying the SAW at the speed of sound decay exponentially with the
distance from the surface on which the wave propagates. As the piezoelectric
and the semiconductor under consideration are usually acoustically mismatched,
no wave is propagating on the semiconductor. This fact makes the theoretical
description quite straight forward: As has been pointed out earlier by
Schenstroem et al. [9] and more recently by Drichko et al. [12], the frequency
dependence of both quantities
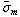and
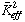can be calculated analytically if one uses some of the above simplifying
approximations. The critical conductivity
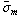results
in
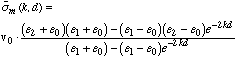(2)
where [[epsilon]]1 and [[epsilon]]2 are the dielectric constants of the
piezoelectric substrate and the semiconductor, respectively. The effective
coupling constant
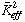can
be expressed by the experimentally observable maximum attenuation :
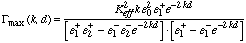(3)
Here, the abbreviation
has been used. Both quantities (2) and (3) depend exponentially on the
parameter kd and on a properly averaged dielectric constant as indicated
by
.
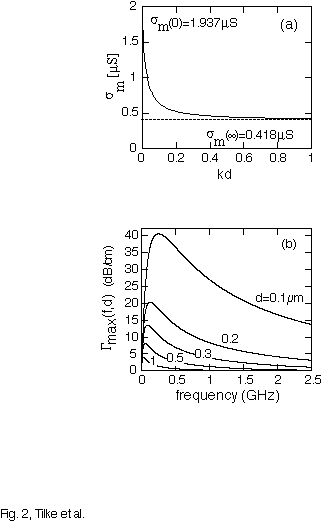
In Fig. 2 we plot the results of eq. (2) for
and for [[Gamma]]max according to eq. (3). The initial increase of [[Gamma]]max
at low frequencies is caused by the proportionality of the attenuation and the
SAW wave vector (cf. eq. 1). For higher frequencies, however, the exponential
influence of the airgap gains importance which finally leads to a strong
decrease of the maximum attenuation [[Gamma]]max. As [[Gamma]]max in a sense
determines the maximum achievable interaction strength, this quantity is very
useful for the estimate of potential experimental or device applications.
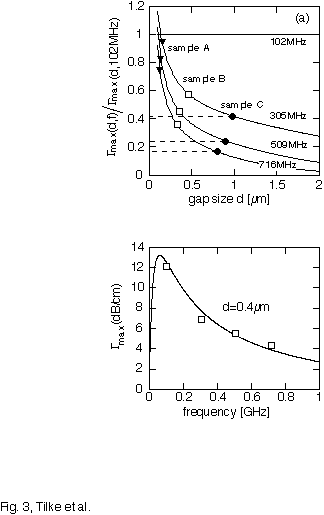
From our measurement of both
and [[Gamma]]max at different frequencies for the same cooling cycle and sample
configuration we are now able to deduce the size of the residual airgap of the
specific experiment. In Fig. 3(a) we show the result of such an evaluation for
three different sandwich systems. For the sake of this experiment, no special
care was taken to reproduce a specific airgap for the three configurations.
According to eq. (3) we plot the expected relative maximum attenuation as a
function of the size of the airgap for different frequencies (solid lines)
together with the actual measurements for different frequencies and the three
different samples (dashed lines). To be able to compare the results, all
theoretical lines have been normalized to the one at f=102 MHz. The crossing
point (symbols) of the dashed and the corresponding solid lines indicates the
average gap size for a given sandwich system. For high frequencies this gap
size seems to be slightly smaller than for lower frequencies which we take as
an indication that we are only able to determine an average size of the gap and
for high frequencies the regions of the active sample area with small gaps
contribute significantly to the interaction. Using this given gap size, the
experimentally obtained maximum attenuation is well described by eq. (3) over
the whole accessible frequency range as it is demonstrated in Fig. 3(b) for
sample B. Also, the frequency dependence of
is
well described by the model as described by eq. (2). Given the gap size d and
using eq. (2) we are able to reproduce (not shown) the size of the splitting
and the actual line shape for split quantum oscillations at high magnetic
fields.
In summary, we investigate the acousto electric interaction between a surface acoustic wave and a quasi-two dimensional electron system in a semiconductor heterostructure as a function of SAW frequency using a proximity coupling technique. The existence of a small residual airgap between the piezoelectric and the semiconductor containing the Q2DES leads to a frequency dependent strength of the interaction that can be described using simple models. We study this frequency dependence experimentally using multi-frequency SAW delay lines and different semiconductor structures. Excellent and quantitative agreement between our experiments and the theoretical model is achieved.
We gratefully acknowledge fruitful discussions with M. Rotter, and J.P. Kotthaus, and thank S. Manus and W. Kurpas for technical support. The SAW delay lines have been kindly provided by Dr. W. Ruile (Siemens AG, Munich). The semiconductor test structures are grown Dr. W. Wegscheider at the Walter-Schottky-Institute in Munich (sample B) and in the group of Prof. Kroemer in Santa Barbara, USA (samples A and C). We acknowledge financial support of the German-Israeli Foundation for Scientific Research (GIF) under contract No. I-328-248.07/93.
References :
1 A. Wixforth, J.P.Kotthaus, and G. Weimann,
Phys. Rev. Lett. 56, 2104 (1986)
see also: A. Wixforth, J. Scriba, M. Wassermeier, J.P. Kotthaus, G. Weimann, and W. Schlapp,
Phys. Rev. B40, 7874 (1989)
2 R.L. Willett, M.A. Paalanen, R.R. Ruel, K.W. West, L.N. Pfeiffer, and D.J. Bishop,
Phys. Rev. Lett. 65, 112 (1990)
3 R.L. Willett, R.R. Ruel, M.A. Paalanen, K.W. West, and L.N. Pfeiffer,
Phys. Rev. B47, 7344 (1993)
4 J.M. Shilton, D.R. Mace, V.I. Talyanskii, Yu Galperin, M.Y. Simmons, M. Pepper, and D.A. Ritchie, J. Phys.: Condens. Matter 8, L337 (1996)
5 R.L. Willett and L.N. Pfeiffer,
Surf. Sci. 361, 38 (1996)
6 A. Esslinger, R.W. Winkler, C. Rocke, A. Wixforth, J.P. Kotthaus, H. Nickel, W.Schlapp, and R. Lösch,
Surf. Sci. 305, 83 (1994)
7 G.R. Nash, S.J. Bending, M. Boero, P. Grambow, K. Eberl, and Y. Kershaw,
Phys. Rev. B54, 8337 (1996)
8 J. M. Shilton, V.I. Talyanskii, M. Pepper, D.A. Ritchie, J.E.F. Frost, C.J.B. Ford, C.G. Smith, and G.A.C. Jones,
J.Phys.:Condens. Matter 8, L531 (1996)
9 A. Wixforth, J. Scriba, M. Wassermeier, and J. P. Kotthaus, G. Weimann, and W. Schlapp,
J. Appl. Phys. 64, 2213-2215 (1988)
10 A. Schenstrøm, Y.J. Qian, M.-F. Xu, H.-P. Baum, M. Levy, and Bimal K. Sarma,
Sol. State Com. 65, 739 (1988)
11 H. Engan,
IEEE Trans. Son. Ultrason., SU-22, 395 (1975)
12 B. Fleischmann, W. Ruile, G. Riha,
Ultrason. Symp. Proc. 1986, p. 163-167
13 I.L Drichko, A.M. Diakonov, V.D. Kagan, A.M. Kreshchuk, G.D.Kipshidze, T.A. Polyanskaya, I.G. Savel'ev, I.YU. Smirnov, and A.V. Suslov,
Phys. Low-Dim. Struct. 10/11, 275 (1995)
Figure captions :
Fig. 1 : Typical SAW transmission experiments for a heterostructure sample in a magnetic field using the proximity coupling scheme and different frequencies. Giant quantum oscillations of the SAW intensity reflect the Shubnikov-deHaas oscillations of the diagonal magnetoconductivity [[sigma]]xx [1]. Both the maximum attenuation [[Gamma]]max as well as the critical conductivity [[sigma]]m decrease with increasing frequency.
Fig. 2 : (a) Theeoretical plot of the critical conductivity [[sigma]]m where maximum attenuation occurs as a function of the product kd. k =2[[pi]]/[[lambda]] denotes the SAW wave vector and d the size of the residual airgap.
(b) Maximum attenuation [[Gamma]]max for a given airgap as a function of the frequency. For high frequencies [[Gamma]]max decreases according to the model calculation.
Fig. 3 : (a) Experimentally obtained normalized maximum attenuation [[Gamma]]max (symbols) for three different frequencies and three different samples together with the theoretical predictions (solid lines). Comparison of theory and experiment results in the average size of the airgap for a given experiment (dashed lines).
(b) Given the airgap of d=0.4um for sample B (Fig.3a), the behavior of the experimentally obtained maximum attenuation as a function of frequency is well described by the model.
